Random isometries
In many applications, including quantum communication and quantum error correction, it is useful to encode a quantum state of one system into a quantum state of a larger system.
Such operations are described by isometries.
You may think about isometries as a generalisation of unitaries: like unitaries, they preserve inner products; unlike unitaries, they are maps between spaces of different dimensions.
Let \mathcal{H} and \mathcal{H}' be Hilbert spaces such that \dim\mathcal{H}\leqslant\dim\mathcal{H}'.
An isometry is a linear map V\colon\mathcal{H}\to\mathcal{H}' such that V^\dagger V=\mathbf{1}_{\mathcal{H}}
Isometries preserve inner products, and therefore also the norm and the metric induced by the norm.
An isometry V\colon\mathcal{H}\to\mathcal{H}' maps the whole Hilbert space \mathcal{H} onto a subspace of \mathcal{H}'.
As a consequence, the matrix representation of an isometry is a rectangular matrix formed by selecting only a few of the columns from a unitary matrix.
For example, given a unitary U we can construct an isometry V as follows:
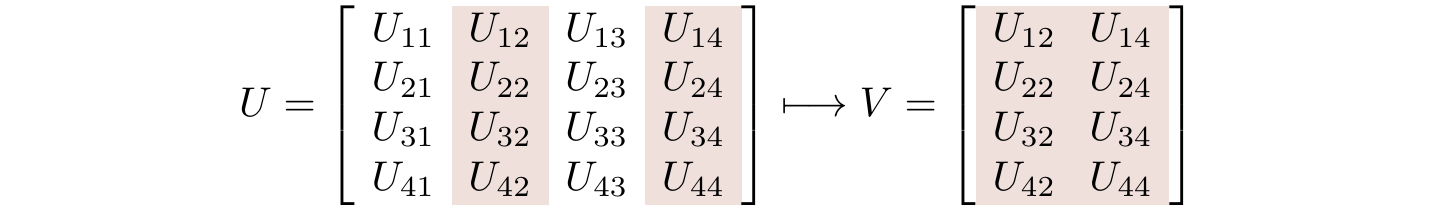
The fact that an isometry V preserves the inner products comes from the fact that we require V^\dagger V=\mathbf{1}_{\mathcal{H}};
we do not require VV^\dagger=\mathbf{1}_{\mathcal{H'}}.
Indeed, if we required both of these, then that would be equivalent to asking for V to be unitary.
The operator VV^\dagger is a projector operator acting on \mathcal{H}', which projects onto the image of \mathcal{H} under the isometry V, as we can see by expressing V in Dirac notation:
V = \sum_i |b_i\rangle\langle a_i|,
where the |a_i\rangle form an orthonormal basis in \mathcal{H}, and the |b_i\rangle are just orthonormal (but not necessarily spanning) vectors in \mathcal{H}';
in the special case where V is unitary, the orthonormal vectors |b_i\rangle form an orthonormal basis in \mathcal{H}'.
Writing V in this form, it is clear that V^\dagger V=\sum_i |a_i\rangle\langle a_i|=\mathbf{1}, and that VV^\dagger = \sum_i |b_i\rangle\langle b_i| projects on the subspace spanned by |b_i\rangle.
Although isometries are strictly more general than unitaries, an fundamentally important fact is that isometries still represent physically admissible operations: they can be implemented by bringing two systems together (via tensoring) and then applying unitary transformations to the composite system.
That is, take some system \mathcal{A} in state |\psi\rangle, and bring in another system \mathcal{B} in some fixed state |b\rangle;
applying some unitary U to the combined system \mathcal{A}\mathcal{B} then gives an isometry from \mathcal{H}=\mathcal{H}_\mathcal{A} to \mathcal{H}'=\mathcal{H}_\mathcal{A}\otimes\mathcal{H}_\mathcal{B}, i.e. the result is a linear map V defined by
V\colon
|\psi\rangle
\longmapsto
|\psi\rangle|b\rangle
\longmapsto
U(|\psi\rangle|b\rangle).
Any isometry is a quantum channel, since any quantum state described by the state vector |\psi\rangle (or by a density operator \rho) is transformed as
|\psi\rangle\longmapsto V|\psi\rangle
(or as \rho\mapsto V\rho V^\dagger), and the normalisation condition is exactly the defining property of isometries:
V^\dagger V =\mathbf{1}.
Isometries are incredibly important when it comes to error correction, and we will see them again much more in Section ??.
